Limits to Growth and the Malthusian Trap
Xavier Timbeau 
Anissa Saumtally
Graphical representation
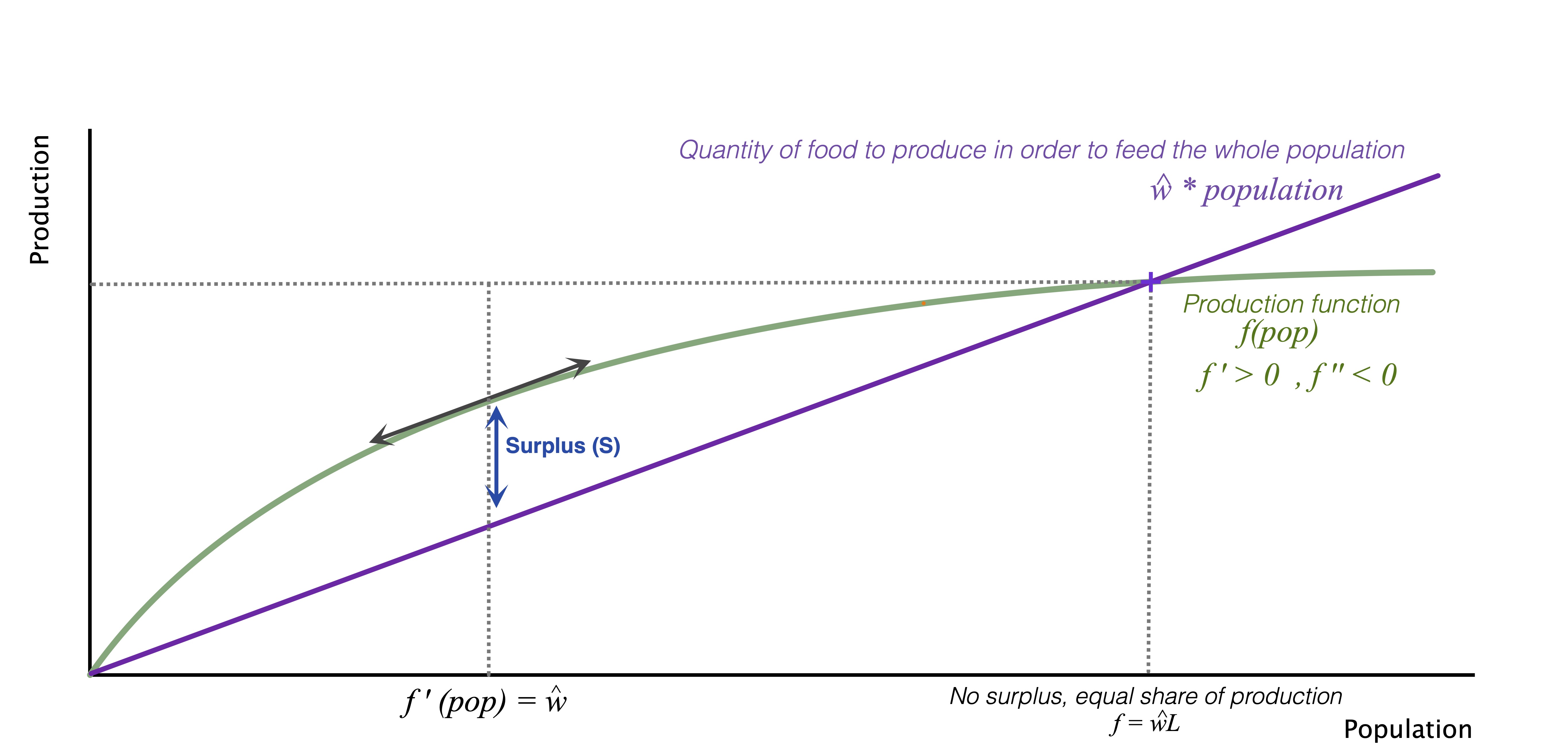
Suppose a usual Cobb-Douglas production function \[f(K,L) = A K^\alpha L^{1-\alpha} , 0 < \alpha < 1 \]
\(K\) stands for capital (i.e. land resources), with a fixed quantity.
\(L\) stands for labour, unit is an individual, and for simplicity \(L\) is equal to the population (no one is inactive)
\(w\) is the wage, with \(\widehat{w}\) the starvation income (minimum income needed to not die)
\(S\) is the surplus : \(S = f - wL\)
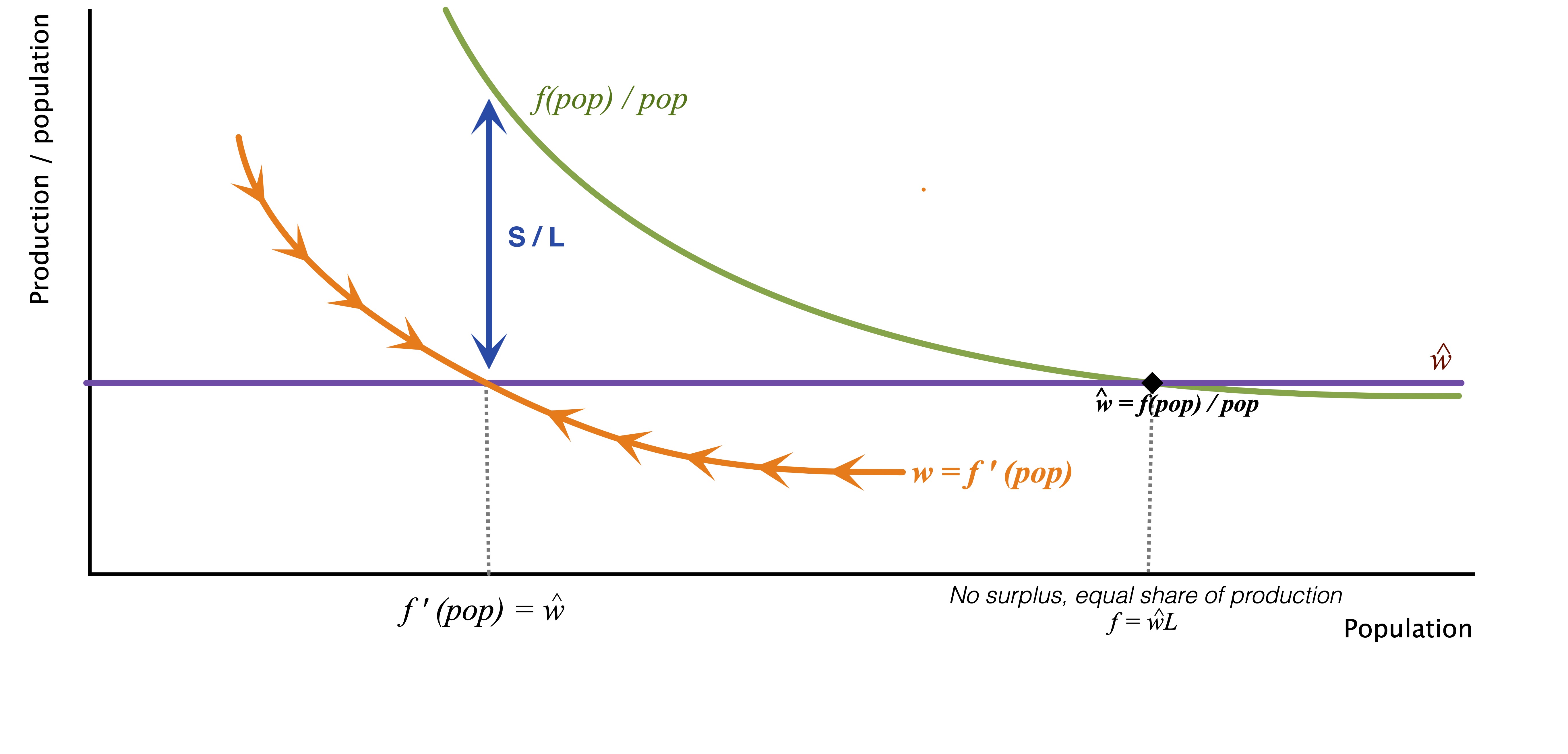
\[\frac{f}{pop} = \frac{f}{L} =\frac{AK^\alpha}{L^\alpha}\] is a decreasing function of \(L\)
In a competitive labour market:
\[w=f_L^\prime= (1 - \alpha) \frac{f}{L} < \frac{f}{L}\] Equilibrium population in a competitive market: \[L^*= \left(\frac{(1−𝛼)𝐴}{\widehat{w}}\right)^{\frac{1}{\alpha}}K\]
Full share equilibrium:
\[L^{**}=\left(\frac{A}{\widehat{w}}\right)^{\frac{1}{\alpha}}K=\frac{L^*}{(1-\alpha)^{\frac{1}{\alpha}}} > L^*\] When \(f_L^\prime = \widehat{w}\) , then \(S = \frac{\alpha}{1-\alpha}\widehat{w}L^* > 0\)
The higher the \(\alpha\), the higher the surplus.
How to observe a change in population
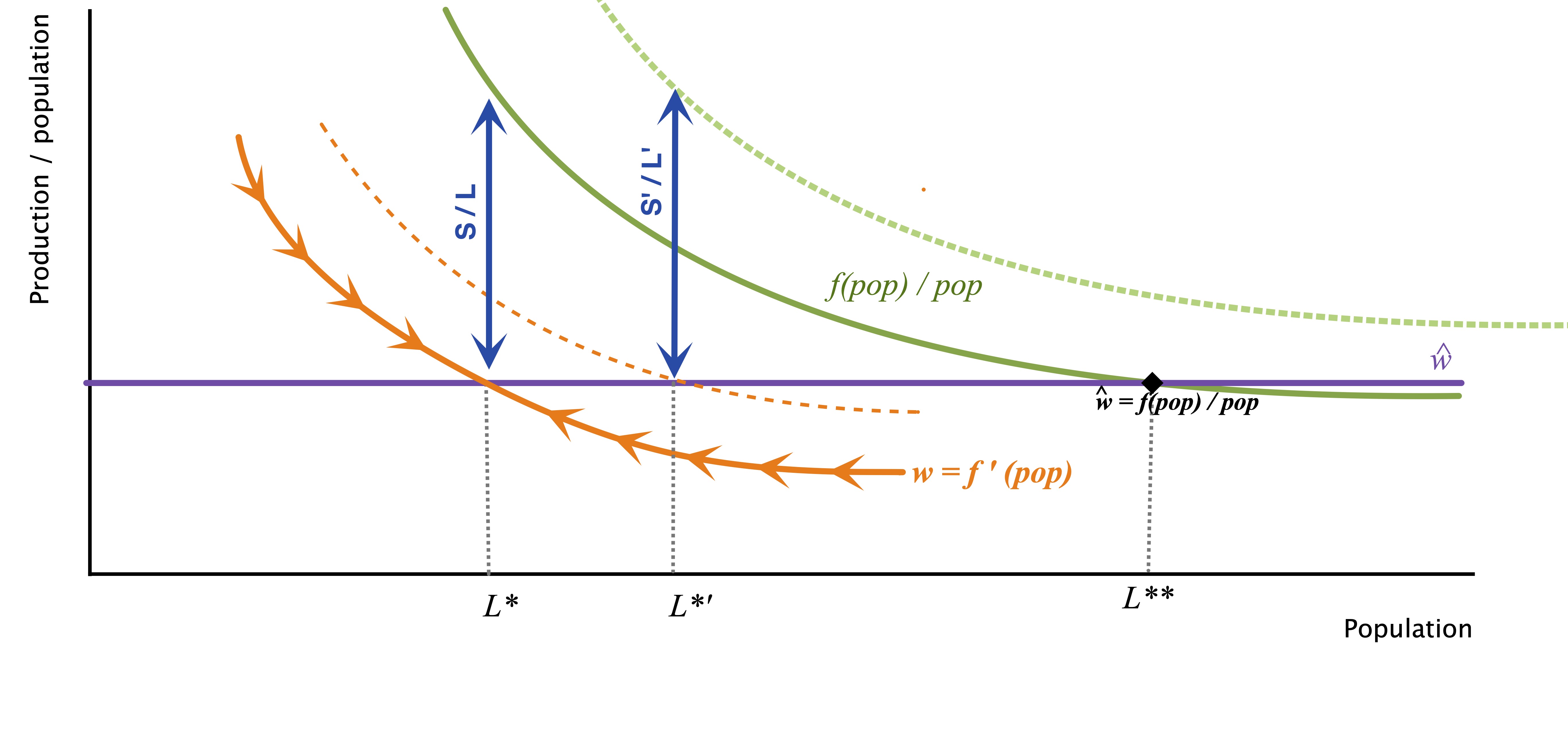
An increase in \(K\) (i.e. more land) or in \(A\) (technical progress) will shift the production function upwards .
- Population is going to increase :
\[L^*= \left(\frac{(1−𝛼)𝐴}{\widehat{w}}\right)^{\frac{1}{\alpha}}K\]
- And surplus is going to increase
\[ S = \frac{\alpha}{1-\alpha}\widehat{w}L^* = \alpha \left(\frac{1-\alpha}{\widehat{w}}\right)^{\frac{1-\alpha}{\alpha}} A^{\frac{1}{\alpha}}K\]
- However, wages remain unchanged (and equal to \(\widehat{w}\))
From property to starvation
Starvation is a fixed point
- Population dynamic is key
- Average product is the sum of marginal product (per head) and surplus (per head). Marginal production per head is equal to starvation income, is median income and is constant (i.e., independent of 𝐾 or of 𝐴)
- The gap between mean and median income is inequality
Property of land implies surplus
- Fixed quantity of land and increasing population means inequality
A “robust” model from 10 000 BC to circa 1750 AD
- Malthus didn’t anticipate exit from the trap
- His only conclusion was population control
No sex before marriage, especially for the poor
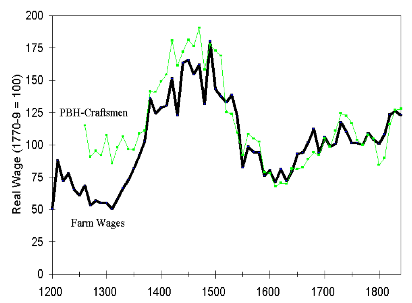
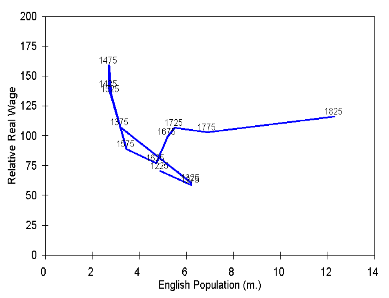
England in the Trap
Source: Clark, G., Rogers, J. E. T., Beveridge, W., Gilboy, E., & Phelps-brown, H. (1850). THE LONG MARCH OF HISTORY : FARM LABORERS ’ WAGES IN ENGLAND 1208-1850, 1–35.
Wages are built using various sources, based on archive data, manuscript wages statement, and so on. Cost of living is based on price information about grains.
Escaping the Malthusian Trap
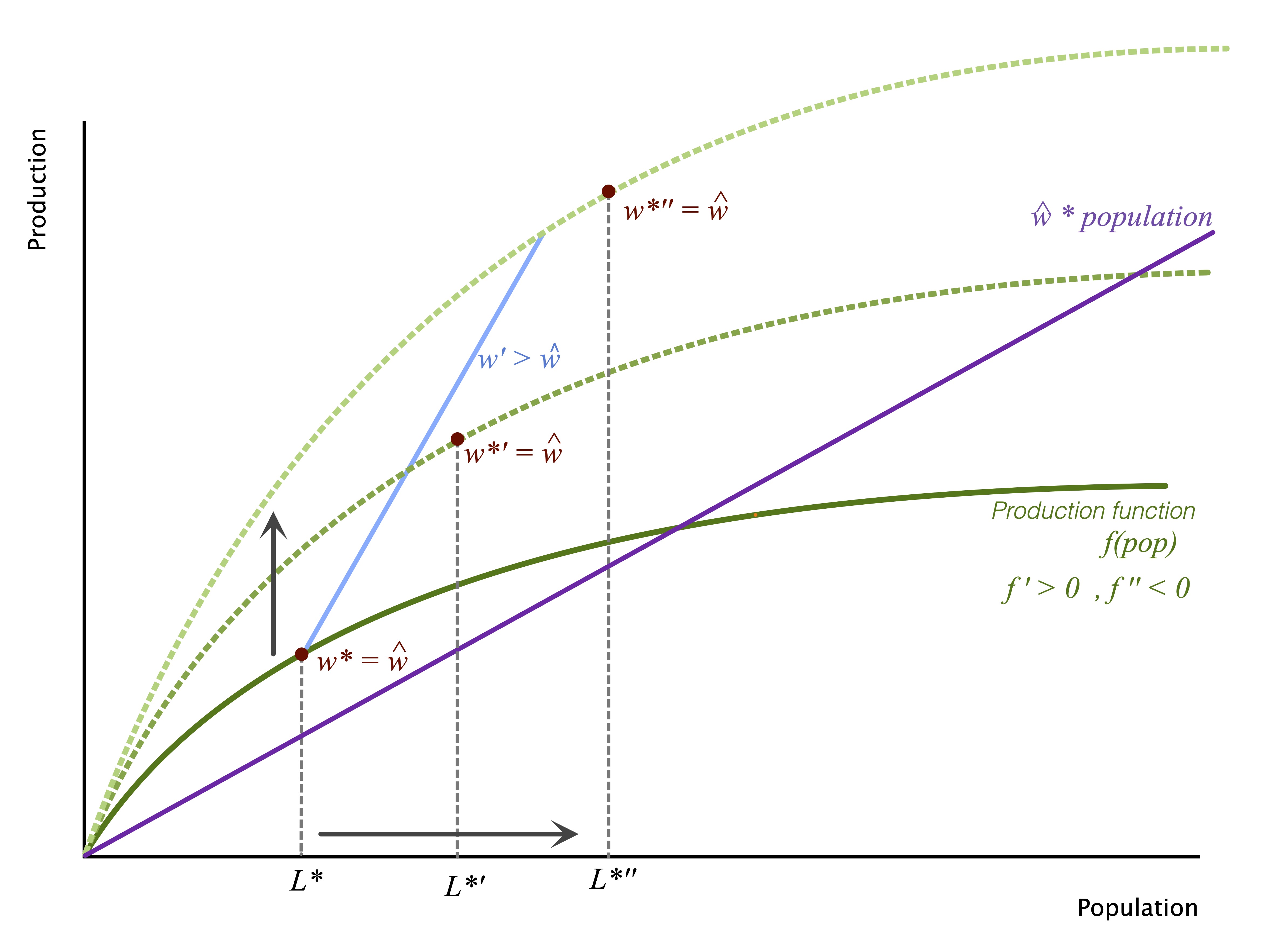
Imagine a production shift “faster” than the increase in population:
- faster means than population does not succeed to reach population equilibrium
Then, population is lagging for equilibrium population
- There is room (through market forces) for wage increase above \(\widehat w\), the starvation income
Then median food/population can rise, and starvation is no more a “point fixe”
Widespread (by the median individual) accumulation is made possible
- Increases individual productivity, participate in shifting the production function, self-sustained dynamics
positive feedback loop #1
Abel and Cain
Source: Tintoretto
The Death of Remus and the end of Pastoralism
The modernity of Malthusian trap
What lessons would you draw from the Malthusian trap model ?
Land may not be scarce in the future, but what else could be ?
What could be done when facing scarcities ?
